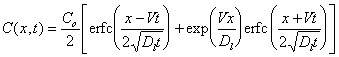
| [TOC] | 4.4 One-Dimensional Conservative Solute with a Continuous Source | [Prev. Page] | [Next Page] |
This section evaluates model performance in simulating one-dimensional transport of a conservative solute with a continuous source. It reports RSSE as a function of Peclet (Pe) and Courant (Co) numbers. The analytical equation representing solute flow in the aquifer is (Fetter, 1994):
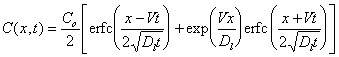 |
(4.5) |
Where: t = time (T) x = distance from inlet (L) Co = concentration at inlet (M/L3) V = pore water velocity (L/T) Dl = dispersion coefficient (L2/T)
Equation (4.5) makes the following assumptions about initial and boundary conditions:
The model is incapable of simulating an aquifer of infinite length. This should not be a problem if the performance tests use simulation times short enough to prevent concentration profiles from reaching the aquifer outlet boundary.
Figure 4.4.1 illustrates log RSSE contours as a function of Peclet (Pe) and Courant (Co) numbers:
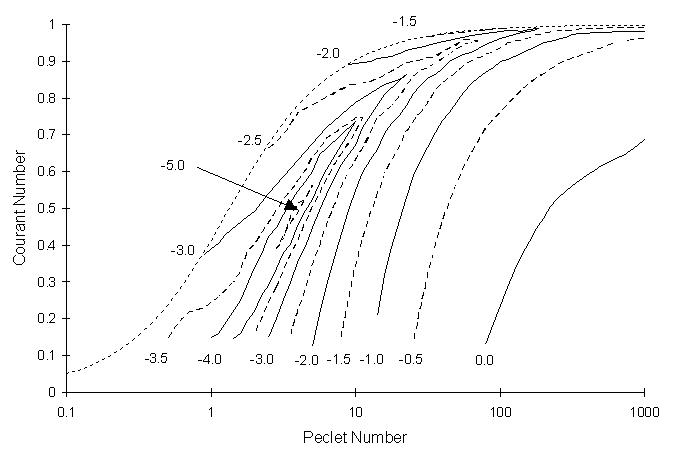
Figure 4.4.1 Log RSSE Contours as a Function of Pe
and Co for One-Dimensional Conservative Solute Transport
with Continuous Source
Figure 4.4.1 shows that the RSSE is highly sensitive to the choice of the Peclet and Courant number. In addition, it shows that the region around Pe » 4.0 and Co » 0.5 contains the lowest RSSE values and falls within the region of no oscillations in Figure 3.8.3.
Figure 4.4.1 also shows that selection of the appropriate Courant number has implications on model performance when simulating multiple solutes or heterogeneous systems. A Courant and Peclet number may be appropriate for one of the solutes and inappropriate for the others. For simulations with heterogeneous flow systems, different Courant and Peclet numbers will occur in different areas of the flow field.
Figure 4.4.2 through Figure 4.4.4 illustrate concentration profiles for various combinations of Peclet and Courant numbers. The three figures illustrate choices of: low Courant and Peclet number; low Courant number and High Peclet number; and high Courant and Peclet number. Circles and lines represent numerical and analytical values respectively.
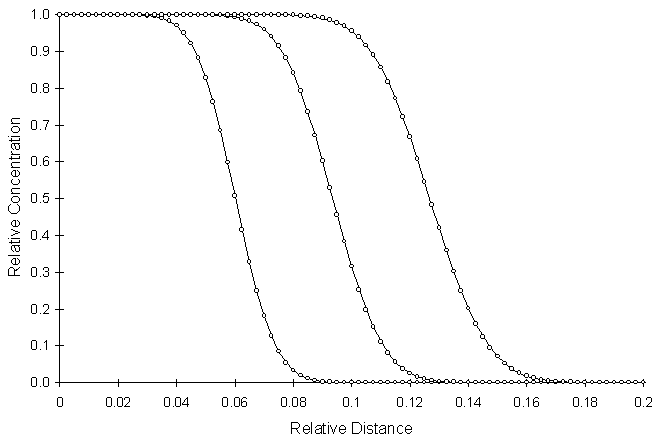
Figure 4.4.2 One-Dimensional Conservative Solute
Transport with Continuous Source, Pe = 2.5, Co =
0.36, RSSE = 1.0 x 10-5
Figure 4.4.2 illustrates the choice of a low Courant and Peclet number. This combination of Courant and Peclet numbers falls in the region of no oscillations in Figure 3.8.3.
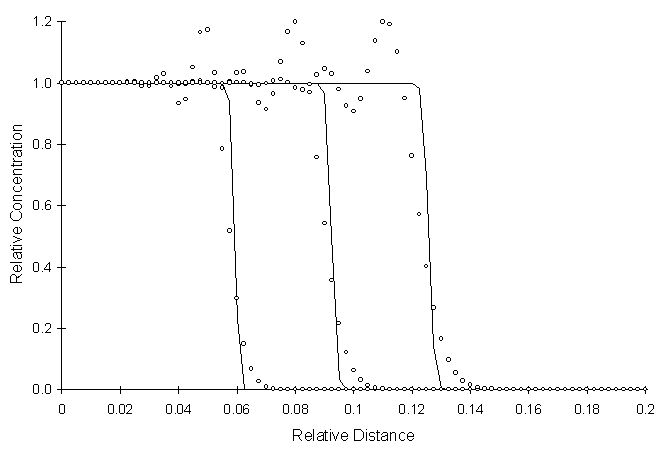
Figure 4.4.3 One-Dimensional Conservative Solute
Transport with Continuous Source, Pe = 250, Co =
0.36, RSSE = 1.2
Figure 4.4.3 illustrates a simulation with space oscillations. The choice of the Courant number is too low for the choice of Peclet number. This combination of Courant and Peclet number falls in the region of space oscillations in Figure 3.8.3.
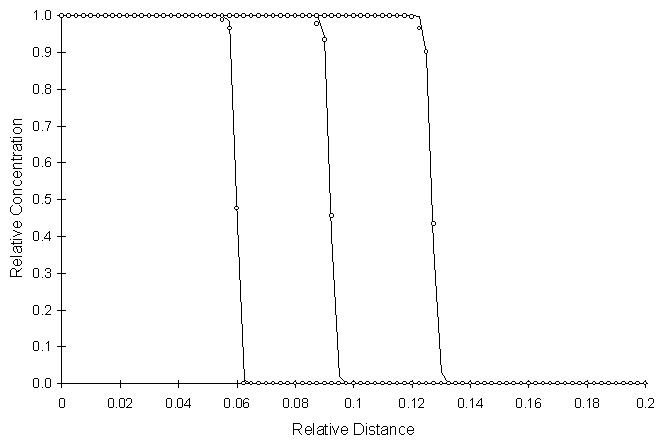
Figure 4.4.4 One-Dimensional Conservative Solute
Transport with Continuous Source, Pe = 250, Co =
0.995, RSSE = 1.1 x 10-2
Figure 4.4.4 illustrates the simulation of highly advective system. The combination of Courant and Peclet number falls in the region of no oscillations.
| [Home] | [Table of Contents] | [Prev. Page] | [Next Page] |
| A Two Dimensional Numerical Model for Simulating the
Movement and Biodegradation of Contaminants in a Saturated Aquifer © Copyright 1996, Jason E. Fabritz. All Rights Reserved. |
|||